Question

Find the 9th and 15th terms of the following geometric sequence 2, -4, 8, -16​

Answer 1

Explanation:

Hey there!

The given geometric sequence is: 2, -4, 8, -16.

The;

a1 = 2

Common ratio (r) = T2/T1

= -4/2

= -2

Now;

Use general formula of geometric sequence;

`tn = {a1.r}^(n - 1)`

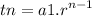
Where, "a1" is first term, "n" is no.of terms and "r" is common ratio.

Then;

`t9 = 2 * { (- 2)}^(9 - 1)`

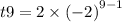
or, t9 = 2*256

Therefore, t9 = 512.

Again;

`t15 = 2. { (- 2)}^(15 - 1)`

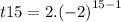
or, t15= 2*16384

Therefore, t15 = 32768.

Hope it helps!

Answer 2

Explanation:

given the geometric sequence 2, -4, 8, -16, ...

a1 = 2

r = -4/2 = -2

find : a9 and a15

solutions:

an = a1. r^(n-1)

=> a9 = 2. (-2)^(9-1)

= 2. (-2)^8

= 2. 2^8

= 2^9

= 512.

=> a15 = 2. (-2)^(15-1)

= 2. (-2)^14

= 2. 2^14

= 2^15

= 32,768