Question

The current population of Fun City is 21000 people. If the population of the city will double every 51 years then the population after 171 years would be

Answer 1

The population of Fun City after 171 years would be 214563.

Explanation:

The statement depicts a case of exponential growth, whose model is described below:

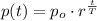`p(t) = p_(o)\cdot r^{(t)/(T) }` (1)

Where:

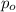`p_(o)` - Initial population, no unit.

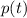`p(t)` - Current population, no unit.

`r` - Growth rate, no unit.

`t` - Time, in years.

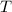`T` - Growth period, in years.

If we know that
`p_(o) = 21000`,
`r = 2`,
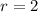`t = 171\,yr` and
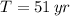`T = 51\,yr`, then the population of the city after 171 years is:

`p(t) = 21000\cdot 2^{(171)/(51) }`

`p(t) = 214563`

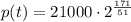
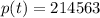
The population of Fun City after 171 years would be 214563.