Answer:
Explanation:
First of all, I'm having a bit of trouble seeing what the exponent on the leading term in the distance is, but I think its an x-squared so that's what I used to solve this. Keep in mind that you do NOT get a number answer here; you get another expression in terms of x for an "answer".
Let's simplify down the rate first by multiplying. What I did was put the denominator under each of the terms in the numerator and then simplified by dividing each term:
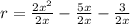 which simplifies to
which simplifies to
 Now let's get rid of that denominator there by multiplying everything by 2x to get
Now let's get rid of that denominator there by multiplying everything by 2x to get
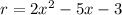 and we're ready to solve for time.
and we're ready to solve for time.
If d = rt, then
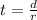 so filling in:
so filling in:
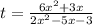 and we can divide this using the method of long division. It would be impossible to show you how to do that here in this forum, but when you divide, you get
and we can divide this using the method of long division. It would be impossible to show you how to do that here in this forum, but when you divide, you get
 ...as crazy as that seems.
...as crazy as that seems.