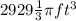Question

What is the volume of sphere with radius 13 ft?

Answer 1

The volume of this sphere is equal to
`2929(1)/(3) \pi ft^(3)`

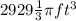
Explanation:

In order to solve this question, we need to know the formula for the volume of a sphere which is...

`V = (4)/(3)\pi r^(3)` ("V" is the volume of the sphere, and "r" is the radius of the sphere)

Now we have to substitute the values that we already know into the formula, and we will get that...

`V = (4)/(3)\pi r^(3)\\\\V = (4)/(3) \pi (13ft)^(3) \\\\V = (4)/(3) \pi (2,197ft^(3) )\\\\V = 2,929(1)/(3) \pi ft^(3)`

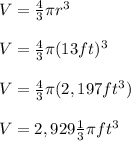
Therefore, the volume of this sphere is equal to
`2929(1)/(3) \pi ft^(3)`

Answer 2

`\displaystyle V = (8788 \pi)/(3) \ ft^3`

General Formulas and Concepts:

Pre-Algebra

Order of Operations: BPEMDAS

1. Brackets
2. Parenthesis
3. Exponents
4. Multiplication
5. Division
6. Addition
7. Subtraction

• Left to Right

Geometry

Volume of a Sphere Formula:
`\displaystyle V = (4 \pi)/(3)r^3`

• r is radius

Explanation:

Step 1: Define

Identify variables

r = 13 ft

Step 2: Find Volume

1. Substitute in variables [Volume of a Sphere Formula]:
   `\displaystyle V = (4 \pi)/(3)(13 \ ft)^3`
2. Evaluate exponents:
   `\displaystyle V = (4 \pi)/(3)(2197 \ ft^3)`
3. Multiply:
   `\displaystyle V = (8788 \pi)/(3) \ ft^3`