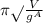Answer: A body of uniform cross-sectional area A and mass density ? floats in a liquid of density ?0
(where ? < ?0), and at equilibrium displaces a volume V. Making use of Archimedes’ principle
(that the buoyancy force acting on a partially submerged body is equal to the mass of the
displaced liquid), show that the period of small amplitude oscillations about the equilibrium
position is
T= 2