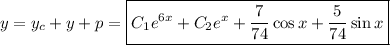Question

Giải phương trình y′′ − 7y′ + 6y = sin x.

Answer 1

Start with the underlying homogeneous equation:

`y''-7y'+6y=0`

which has characteristic equation

`r^2-7r+6=(r-6)(r-1)=0`

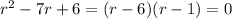
with roots at r = 6 and r = 1. So the characteristic solution is

`y_c=C_1e^(6x)+C_2e^x`

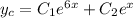
Now for the particular solution, we can use the method of undetermined coefficients, with the following ansatz (the "guess" solution) and its derivatives,

`y_p=a\cos x+b\sin x`

`{y_p}'=-a\sin x+b\cos x`

`{y_p}''=-a\cos x-b\sin x`

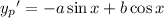
Substituting these into the original equation gives

`(-a\cos x-b\sin x)-7(-a\sin x+b\cos x)+6(a\cos x+b\sin x)=\sin x`

`(5a-7b)\cos x+(7a+5b)\sin x=\sin x`

`\implies\begin{cases}5a-7b=0\\7a+5b=1\end{cases}\implies a=\frac7{74},b=\frac5{74}`

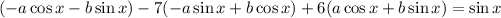
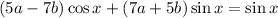
So the particular solution is

`y_p=\frac7{74}\cos x+\frac5{74}\sin x`

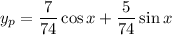
and hence the general solution is

`y=y_c+y+p=\boxed{C_1e^(6x)+C_2e^x+\frac7{74}\cos x+\frac5{74}\sin x}`