Answer:
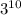
Explanation:
Ok, I gotchu. So, basically, you can do this one of two ways: guess until you get it right or solve with algebra. I am gonna show you the algebra way:
First, let's check to see what 9×9×9×9×9 is. It is 59049
9×9×9×9×9 can be rewritten as
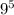 right?
right?
Ok, so now we have to address the main issue here: the problem wants us to give the exponential form but with base 3. To do this, we must find out what power turns 3 into 9. In other words
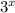 =9. "x" in this case is 2 right? because
=9. "x" in this case is 2 right? because
 is 9.
is 9.
Alright, so we understand that it takes five 9s multiplied by each other (
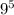 ) to reach 59049 and it takes two 3s multiplied by each other (
) to reach 59049 and it takes two 3s multiplied by each other (
 ) to reach 9. For a visual:
) to reach 9. For a visual:
9 x. 9. x. 9. x. 9. x. 9.
is
 . x.
. x.
 . x.
. x.
 . x.
. x.
 . x.
. x.

So now just evaluate:
 . x.
. x.
 . x.
. x.
 . x.
. x.
 . x.
. x.

When multiplying exponents that have the same base, you leave the base alone and add the exponents. For example:
 x.
x.
 =
=
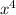
So here we just keep our base of 3 and add up all the 2s
so 2+2+2+2+2=10
That leaves us with our answer:
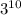 .
.
I hope this helps :). Let me know if you need me to explain anything further.