Question

Without using a calculator, find the exact value of the expression: 9tan2(π/3​)+12sec2(π/4​).

Answer 1

The exact value of the expression 9tan2(π/3)+12sec2(π/4) is found by substituting the exact trigonometric values for tan(π/3) and sec(π/4), which gives us 27 + 24, resulting in an exact value of 51.

Step-by-step explanation:

The question asks us to find the exact value of the expression 9tan2(π/3)+12sec2(π/4) without using a calculator. We can solve this by recalling the exact values for the trigonometric functions at these angles:

tan(π/3) = √3

sec(π/4) = √2 or 1/cos(π/4) since cos(π/4) = √2/2

Now we substitute the exact values into the expression:

9tan2(π/3) = 9(√3)2 = 9*3 = 27

12sec2(π/4) = 12(√2)2 = 12*2 = 24

Adding these values together:

27 + 24 = 51.

So, the exact value of the expression is 51.

Answer 2

To find the exact value of the given expression without using a calculator, we simplify each term using trigonometric identities and then add them together. The result is 51.

Step-by-step explanation:

To find the exact value of the expression 9tan2(π/3)+12sec2(π/4), we need to use trigonometric identities to simplify each term.

First, let's simplify the term 9tan2(π/3). Since tan(π/3) =
`√(3)`, we have:

9tan2(π/3) = 9(
`√(3)`)2 = 9 * 3 = 27.

Next, let's simplify the term 12sec2(π/4). Since sec(π/4) =
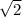`√(2)`, we have:

12sec2(π/4) = 12(
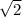`√(2)` )2 = 12 * 2 = 24.

Finally, we can add the simplified terms:

27 + 24 = 51.

Therefore, the exact value of the expression is 51.