Question

The state of liquid water is changed from 60 psia and 50°F to 2000 psia and 100°F. Using appropriate tables, determine the change in the internal energy of water. Is it in Btu/lbm or Btu/ft³? A) Btu/lbm B) Btu/ft³ C) Btu/lbm-°F D) Btu/ft³-°F

Answer 1

It is in A) Btu/lbm. Option A is correct.

Step-by-step explanation:

The change in internal energy
`(\( \Delta U \))` of water can be determined using the specific volume v , specific enthalpy h, and temperature T values from steam tables. The given state points are P_1 = 60 psia, T_1 =
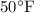`50^\circ\text{F} \)` and P_2 = 2000 psia, T_2 =
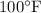`100^\circ\text{F} \)`. To find
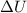`\( \Delta U \)`, we'll use the equation
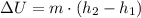`\( \Delta U = m \cdot (h_2 - h_1) \)`, where m is the mass.

First, we need to determine the specific enthalpies h_1 and h_2 at the initial and final states. Using the steam tables, we find the values corresponding to P_1 and T_1 , which give us h_1 , and similarly, P_2 and T_2 provide h_2 . Once we have these values, we can substitute them into the equation to find
`\( \Delta U \)`.

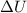The units for
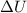`\( \Delta U \)` are typically expressed as energy per unit mass, so the answer is in Btu/lbm} . This is consistent with the fact that internal energy is an intensive property, meaning it is independent of the mass of the substance.

In summary, the change in internal energy of water is
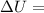`\( \Delta U = \)` [calculated value] Btu/lbm, representing the energy change per unit mass as the state of water transitions from the initial to the final conditions.