Question

Find the area and perimeter.

A.63x^2 + 16; 22x + 14
B.18x^2 + 63x; 16x + 2
C.18x^2 + 63x; 22x + 14
D.63x^2 + 18; 11x + 7

Answer 1

Hello!

Answer:

The answer is C.

`\Large \boxed{ \sf P =14 + 22x}`

`\Large \boxed{ \sf A= 18x^2 + 63x}`

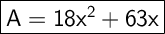
Explanation:

→ We want to calculate the area and the perimeter of this rectangle:

I I
I I 2x + 7
I I
I I
I I

9x

→ We know that:

- the perimeter is equal to
`\sf P = 2(l+w)`

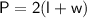
- the area is equal to
`\sf A = l * w`

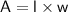
Where:

- P is the perimeter

- A is the area

- l is the lenght

- w is the width

→ Let's apply these formula:

`\sf P = 2(l+w) \\\\= 2(2x+7 + 9x)\\\\= 2(7+11x)\\\\\boxed{ \sf= 14 + 22x}`

`\sf A = l * w\\\\= (2x+7) * 9x\\\\= 9x(2x+7)\\\\\boxed{ \sf = 18x^2 + 63x}`

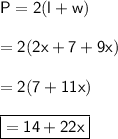
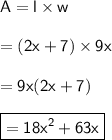
Conclusion:

The perimeter is equal to 14 + 22x.

The area is equal to 18x² + 63x.

→ So the correct answer is C.