Question

The equation (2x+1)^2= -2 has ___ real solutions

Answer 1

Answer: Zero

Explanation:

To find how many real solutions this equation has, we will solve.

Given:

(2x + 1)² = -2

Square root of both sides of the equation:

2x + 1 = ±
`√(-2)`

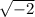
Subtract 1 from both sides of the equation:

2x = ±
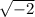`√(-2)` - 1

Divide both sides of the equation by 2;

x = ±
`(√(-2)-1 )/(2)`

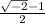
Simplify the square root:

x = ±
`(i√(2)-1 )/(2)`

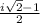
There are no real solutions to this equation.