Question

Which statement about the figure MUST be true? Select all that apply.

Answer 1

○=> Correct options :

□
`\color{plum}\tt\bold{(C) \: SR = 27}`

□
`\color{plum}\tt\bold{(D) \: QR = 54 }`

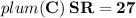
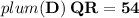
Steps to derive the correct options :

Since two sides and one included angle is equal in △PQS and △PRS, we can conclude that they are congruent under the SAS congruence criterion.

Which means :

▪︎Angle S = Angle S

▪︎PS = PS

▪︎QS = RS

Given :

Measure of segment QS = 6n+3

Measure of segment RS = 4n+11

Thus :

`= \tt6n + 3 = 4n + 11`

`= \tt6n + 3 - 4n = 11`

`=\tt 2n + 3 = 11`

`= \tt2n = 11 - 3`

`= \tt2n = 8`

`=\tt n = (8)/(2)`

`\hookrightarrow\color{plum}\tt n = 4`

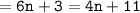
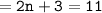
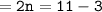
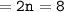
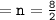
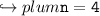
Thus, the value of n = 4

Measure of segment QS :

`=\tt 6n + 3`

`= \tt6 * 4 + 3`

`= \tt24 + 3`

`\color{plum}\tt \: \bold{QS = 6n + 3 = \tt\bold{27}}`

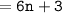
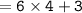
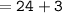
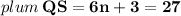
Thus, measure of QS = 27

Measure of RS :

`=\tt 4n + 11`

`=\tt 4 × 4 + 11`

`=\tt 16+11`

`\color{plum}\tt \: \bold{RS = 4n + 11 = \tt\bold{27}}`

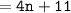
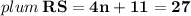
Measure of QR :

`=\tt 27+27`

`\color{plum}\tt \: \bold{QR = 27+27 \tt\bold{=54}}`

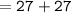
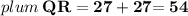
Thus :

▪︎QS = 27

▪︎RS = 27

▪︎QR = 54

Therefore, the correct options are :

▪︎(C) SR = 27

▪︎(D) QR = 54