Question

Name the property of real numbers illustrated by the following equation. three times the quantity of the square root of two plus six, equals three times the square root of two, plus eighteen (1 point) responses closure property closure property associative property of multiplication associative property of multiplication distributive property distributive property commutative property of multiplication

Answer 1

The equation involves the distributive property, where a number outside parentheses is distributed across the terms within parentheses and multiplied with each separately.

Step-by-step explanation:

The property of real numbers illustrated by the equation three times the quantity of the square root of two plus six, equals three times the square root of two, plus eighteen is the distributive property. The distributive property states that multiplication distributed over addition or subtraction remains valid. In the given equation, when we distribute the three over the sum inside the brackets, we multiply both the square root of two and six by three separately, resulting in the simplified expression on the right side of the equation.

Answer 2

The property of real numbers illustrated by the given equation is the distributive property.

Step-by-step explanation:

In the given equation, we have
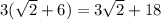`\(3(√(2) + 6) = 3√(2) + 18\)`. This can be understood through the distributive property, which states that for any real numbers (a), (b), and (c), (a(b + c) = ab + ac). In our equation, (a = 3),
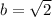`\(b = √(2)\)`, and (c = 6).

Now, applying the distributive property:

`\[3(√(2) + 6) = 3 \cdot √(2) + 3 \cdot 6 = 3√(2) + 18.\]`

This confirms that the left side of the equation is equal to the right side, validating the use of the distributive property.

The distributive property is a fundamental property of real numbers that allows us to distribute a factor across the terms inside parentheses. It plays a crucial role in simplifying expressions and solving equations. In this case, it enables us to break down the multiplication of 3 by the sum of
`\(√(2)\)` and 6, leading to the concise and equivalent expression on the right side. The distributive property is a foundational concept in algebra, providing a systematic way to manipulate expressions and equations in mathematics.