The table allows us to estimate the solution without complex calculations, and x = 0.5 is the closest approximation based on the available data.
The row closest to the actual solution is the one where the values in the second and third columns are closest to each other.
Understanding the Equation:
The equation √(2x + 3) = x/(x + 5) + 2 is non-linear, meaning it cannot be solved using simple algebraic techniques.
The table provides approximate values for both sides of the equation for different values of x.
Our goal is to find the row where these values are closest, indicating a potential solution.
Analyzing the Table:
We're looking for rows where the difference between the values in the second and third columns is as small as possible.
Examining the table, we see that the values are closest in the row where x = 0.5.
Interpreting the Results:
In the row where x = 0.5:
The value of √(2x + 3) is approximately 2.
The value of x/(x + 5) + 2 is approximately 2.0909.
These values are closer to each other than in any other row, suggesting that x = 0.5 is the closest approximation to the actual solution.
Importance of Approximation:
It's crucial to remember that the table provides approximations, not exact values.
Graphically plotting the two expressions would further confirm that x ≈ 0.5 is a good approximation of the solution.
Complete Question :
Consider the following equation.
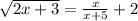
Which row in the table is closest to the actual solution?