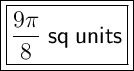
Answer:
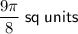
Explanation:
To find the area of the sector, use the area of a sector formula (where the central angle is measured in radians).
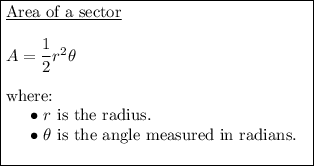
From inspection of the given diagram, the radius of the circle is 3 units, and the central angle of the sector is π/4 radians. Therefore:
Substitute the values of r and θ into the formula, and solve for area (A):
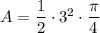
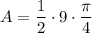
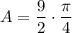
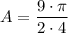
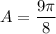
Therefore, the area of the sector is: