-------------------------------------------------------------------------------------------------------------
Answer:

-------------------------------------------------------------------------------------------------------------
Given:
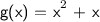
Find:
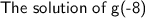
Solution: In order to start working toward the solution, we need to initially set the value of x in the equation to -8.
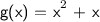
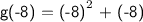
Now, that we have replaced the x variable with -8, we can now simplify the values and combine them to get the final solution. The first number,
 is the same as
is the same as
 which turns out to be 64. We can now subtract 8 from 64 to get our final answer of 56.
which turns out to be 64. We can now subtract 8 from 64 to get our final answer of 56.
Therefore, the final answer is
