Question

A round balloon is filled with a gas. If the balloon has a diameter of 46 cm and is filled with a gas that has a mass of 46 grams. What is the density of the gas?

Answer 1

Approximately
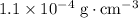`1.1* 10^(-4)\; {\rm g\cdot cm^(-3)}` assuming that the thickness of the balloon is negligible, and that the effect of buoyancy is negligible when measuring the mass of the gas.

Step-by-step explanation:

The density of this gas can be found in the following steps:

• Find the volume of the balloon from its diameter.
• Divide mass by volume to find density.

The volume of a sphere of radius
`r` is
`V = (4/3)\, \pi\, r^(3)`.

For the balloon in this question,
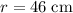`r = 46\; {\rm cm}`. The volume of this balloon would be:

`\begin{aligned} V &= (4)/(3)\, \pi\, (46)^(3) \approx 4.0772 * 10^(5)\; {\rm cm^(3)\end{aligned}`.

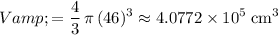Assume that the thickness of this balloon is negligible, such that the volume of the gas inside is equal to the volume of the balloon. Also assume that the mass of the air inside the balloon is measured in a vacuum (such that the effect of buoyancy in the air is negligible.)

To find the density of this gas, divide the mass of this gas by the volume of this gas:

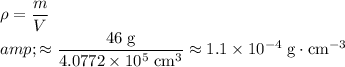`\displaystyle \rho = (m)/(V) \\ &\approx \frac{46\; {\rm g}}{4.0772 * 10^(5)\; {\rm cm^(3)}} \approx 1.1 * 10^(-4)\; {\rm g\cdot cm^(-3)}`.