Question

A bicycle is traveling with a speed of 12 m/s. The cyclist hits the brakes reducing the speed to 4 m/s in a time of 4.8 seconds. What is the acceleration of bike?

Answer 1

So, the acceleration of the bicycle is approximately -1.67 m/s² or it can be said to be decelerating approximately 1.67 m/s².

Introduction

Hi ! Here I will help material about linear motion changes regularly, which is where you will hear a lot of the term acceleration. Acceleration occurs when an object's speed increases in a certain time interval. Acceleration can be negative which is called deceleration. The relationship between acceleration with velocity and time is manifested in the equation:

`\boxed{\sf{\bold{a = (v_2 - v_1)/(t)}}}`

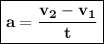
With the following conditions :

• a = acceleration (m/s²)
• 
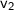  `\sf{v_2}` = object's final velocity (m/s)
• 
  `\sf{v_1}` = object's initial velocity (m/s)
• t = interval of the time (s)

Problem Solving

We know that :

• 
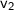  `\sf{v_2}` = object's final velocity = 4 m/s
• 
  `\sf{v_1}` = object's initial velocity = 12 m/s
• t = interval of the time = 4.8 s

What was asked :

• a = acceleration = ... m/s²

Step by step :

`\sf{a = (v_2 - v_1)/(t)}`

`\sf{a = (4 - 12)/(4.8)}`

`\sf{a = (-8)/(4.8)}`

`\boxed{\sf{a \approx -1.67 \: m/s^2}}`

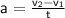
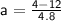
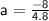
So, the acceleration of the bicycle is about -1.67 m/s² or it can be said to be decelerating around 1.67 m/s².