Answer:
The electrician's income on Tuesday is Rs 2,700
Explanation:
Note:
The median is the middle number of a sorted distribution.
It is the value that divides the distribution into two equal halves, with half of the values being higher than the median and half being lower.
For the Question:
Let's denote the electrician's income on Sunday, Monday, and Tuesday as S, M, and T, respectively.
We are given two pieces of information:
The average income on Sunday and Monday is Rs 1,800:
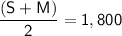
Multiply both sides by 2.

![\sf (S+M) =3,600 ..........[i]](https://img.qammunity.org/2024/formulas/mathematics/college/lgmh11go6akssve0edh94g118thzdut8u1.png)
If his income on Tuesday is also included, the average income will be Rs 2,100:
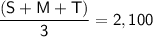
Multiply both sides by 3.
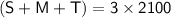
![\sf (S+M+T) =6,300 ..........[i]](https://img.qammunity.org/2024/formulas/mathematics/college/f6pwi0zywd0n7r1jpm7koqz93jcph5qa1c.png)
Substitute this value (S+M) into the second equation:

Subtract 3,600 from both sides:

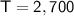
So, the electrician's income on Tuesday is Rs 2,700.