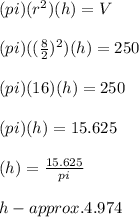Question

Help!!!!! Also shoe the work plz!

Answer 1

`h=(15.625)/(pi)`

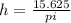
h ~ 4.974

Explanation:

The formula for the volume of a cylinder is;

`(pi)(r^(2))(h)=V`

Where
`(pi)` represents the value,
`3.1415...`

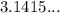
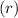`(r)` represents the radius of the given cylinder. In other words, if one were to take a base (the flat side in the case of the cylinder) of the figure. The distance from the center to a point on the outside of the circle is the radius.

`(h)` represents the height of the object. In other words the distance between the two bases of the cylinder.

`V` represents the volume, in other words, the space that the object takes up.

In this problem, one is given the diameter of the cylinder and the volume. One is asked to find the area.

The diameter of a circle is the distance between two opposite sides of the circle. The diameter will always pass through the center of the circle. To find the radius when given the diameter, one will divide by 2.

To solve this problem, one will substitute in the given information and solve for the height using inverse operations.

`(pi)(r^(2))(h)=V\\\\(pi)(((8)/(2))^(2))(h)=250\\\\(pi)(16)(h)=250\\\\(pi)(h)=15.625\\\\(h) = (15.625)/(pi)\\\\h- approx. 4.974`