Question

I need help with these questions?

Answer 1

Hope this helps (it's only thr 1st one tho)
Have a great day, remember to stay hydrated!! :))

Answer 2

`(2)/((x-1)x(x+1))`,
`(y^4)/(8)`, and
`(2)/(3t)`

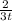
Explanation:

1.

Let's find a common denominator:

`(x(x+1))/((x-1)x(x+1)) - (2(x-1)(x+1))/(x(x-1)(x+1)) + ((x-1)x)/((x+1)(x-1)x)`

`(x(x+1) - 2(x-1)(x+1)+(x-1)x)/((x-1)x(x+1))`

=
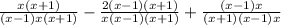
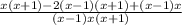
`(2)/((x-1)x(x+1))`

2/(x-1)x(x+1)

2.

`((2y^2)^3)/((8y)^2)`

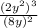
Distribute exponents:

2^3(y^2)^3

__________

8^2(y)^2

Numerator:

2 to the power of 3 equals (2 · 2 · 2) = 8

y to the power of 2 to the power of 3 equals, y to the power of 6 (this is because when exponents in parenthesis are held to the power of another number outside of the parenthesis, you multiply them)

Now let's simplify the denominator:

8 to the power of 2 equals (8 · 8) = 64

y to the power of 2 is just y to the power of 2, so our new expression is:

`(8y^6)/(64y^2)`

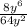
Let's cancel the terms further:

8/64 can be simplified to 1/8

And when you cancel exponents, you subtract the denominator's exponent from the numerator's exponent (
`y^(6-2)` =
`y^(4)`)

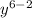Now put them together:

`(1(y^4))/(8)`

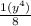
Which is,

=
`(y^4)/(8)`

y^4/8

3.

`(t+2)/(3t) - (1)/(3)`

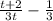
The common denominator for both fractions is 3t, so let's make -1/3's denominator 3t by multiplying t to both the numerator and the denominator:

-1 · t

_____ = -1(t)/3t = -t/3t

3 · t

Now let's solve for the numerator:

`(t+2)/(3t) - (t)/(3t)`

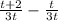
(t + 2) - t, the t's cancel out, t - t + 2 = 2

And the denominator stays the same, so it will still equal 3t

Finally, you'll get an answer of

=
`(2)/(3t)`

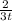
2/3^2