Question

Radical 45g^3h^6 simple

Answer 1

Answer:
`\[3\sqrt[3]{3} * \sqrt[3]{5} * g * h^2\]`

Explanation:

To simplify this, we can break it down into prime factors and then take the cube root of each part:

1. Factorize the numerical part:
`\(45 = 3^2 * 5\)`.

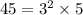2. Break down the variable parts:
`\(g^3\) and \(h^6\)`.

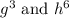3. Take the cube root of each part:

`\[\sqrt[3]{45g^3h^6} = \sqrt[3]{3^2 * 5 * g^3 * h^6} = \sqrt[3]{3^2} * \sqrt[3]{5} * \sqrt[3]{g^3} * \sqrt[3]{h^6} = 3\sqrt[3]{3} * \sqrt[3]{5} * g * h^2\]`

So the simplified expression is:

`\[3\sqrt[3]{3} * \sqrt[3]{5} * g * h^2\]`