Hello!
Answer:
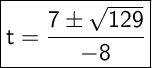
Explanation:
→ We want to solve this equation:
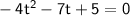
→ It's a quadratic equation because it's on the form:
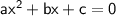
→ Let's use the quadratic formula:
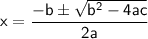
→ In our equation:
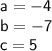
→ Let's apply the quadratic formula:
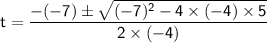
→ Simplify the equation:
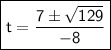
Conclusion:
The solution of the equation
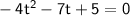 is
is
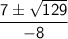 .
.