1 Answer

Jonas Schnelli · Answer 1 · 2024-10-09T22:54:49+0000

Answer:

60°, 60° and 120°.

Explanation:

ABCD is a parallelogram in which one angle is 120°.

We know that,

Let us take
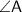$\sf \angle A$ is 120°

As
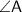$\sf \angle A$ and
$\sf \angle D$ are the opposite angles so they will be equal.

$\sf \angle D$ = 120°

Sum of all the angles of Parallelogram is 360°.

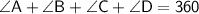
$\sf \angle A +\angle B +\angle C + \angle D = 360\degree$

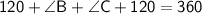
$\sf 120 + \angle B +\angle C + 120\degree = 360\degree$

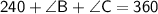
$\sf 240 + \angle B + \angle C = 360\degree$

$\sf \angle B + \angle C = 360 - 240$

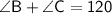
$\sf \angle B + \angle C = 120\degree$

$\sf \angle$ B and
$\sf \angle$ C are also the opposite angles so they will be equal. Let us consider
$\sf \angle$ B and
$\sf \angle$ C be x

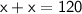
$\sf x + x = 120$

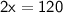
$\sf 2x = 120$

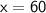
$\sf x = 60$

Hence,

$\sf \angle B$ = 60°

$\sf \angle C$ = 60°

Therefore, the other three angles of the Parallelogram are 60°, 60° and 120°.

___________________