Answer:
x = 53°
Explanation:
Before finding the error, let's know the properties of rhombus.
- All sides are equal.
- Opposite sides are parallel.
- Opposite angles are equal.
- Diagonals bisect each other at right angles.
- Diagonals bisect the angles of a rhombus.
- The sum of two adjacent angles is equal to 180 degrees.
In this case:
Let's the point of intersection of diagonal be O.
m∡ QRP = x
m∡ SQR = 37°
Since diagonals bisect each other at right angles.
So, we have m∡ QOR.
In right angled triangle QOR
m∡ QRP + m∡ SQR + m∡ QOR = 180°
Substitute the value.
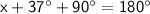

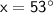
Therefore, Value of x is 53°.