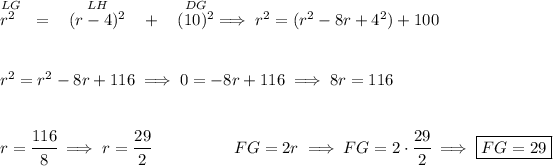Question

Could i please get a step-by step tutorial on this. I know the property of

When two chords of a circle intersect, the product of the lengths of the
segments from one chord equals the product of the lengths of the segments
from the other chord. But im not sure how to figure this question out.

Answer 1

Check the picture below.

since we know the line IG crosses the center of the circle and is perpendicular to the chord, that means the line IG is a bisector of the chord and thus it cuts it in two 10 and 10 segments, as you see there in green.

Now, let's call the radius of the circle "r" and if we subtract GH from the radius "r" we're left with "r - 4", now, let's apply the pythagorean theorem to that, to get the radius, keeping in mind FG = 2r, because FG is a diametrical line.

`\stackrel{ LG }{r^2~}~ = ~~\stackrel{ LH }{(r-4)^2}~~ + ~~\stackrel{ DG }{(10)^2}\implies r^2=(r^2-8r+4^2)+100 \\\\\\ r^2=r^2-8r+116\implies 0=-8r+116\implies 8r=116 \\\\\\ r=\cfrac{116}{8}\implies r=\cfrac{29}{2}\hspace{5em}FG=2r\implies FG=2\cdot \cfrac{29}{2}\implies \boxed{FG=29}`