The vertex of the quadratic equation is (-5, -7).
Solving quadratic equation using completing the square.
The given quadratic equation y = 3x² + 30x + 68. The first step 1 was to separate non x-terms from x-terms. i.e.
y - 68 = 3x² + 30x
Step 2: Divide off the coefficient of x².
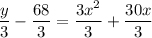
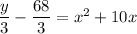
Step 3: Identify the value that completes the square.
Step 4: Complete the square:
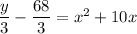
Add 25 to both sides to complete the perfect square trinomial;
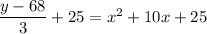
Step 5: Factor and start solving for y.
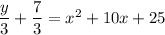
Factor the perfect square trinomial, and begin solving for y.
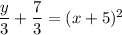
Step 6: Finish solving for y by multiplying both sides by 3.
y + 7 = 3(x + 5)²
Subtract 7 from both sides.
y = 3(x + 5)² - 7
Using the general formula for vertex y = a(x - h)² + k, so the vertex form for the quadratic equation is y = 3(x + 5)² - 7 and the vertex (h,k) is (-5, -7).