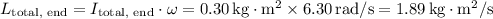Question

A 2.0 m measuring stick of mass 0.225 kg is resting on a table. A mass of 0.500 kg is attached to the stick at a distance of 60.0 cm from the center. Both the stick and the table surface are frictionless. The stick rotates with an angular speed of 6.30 rad/s. (a) If the stick is pivoted about an axis perpendicular to the table and passing through its center, what is the angular momentum of the system? kg: m m?/ (b) If the stick is pivoted about an axis perpendicular to it and at the end that is furthest from the attached mass, and it rotates with the same angular speed as before, what is the angular momentum of the system?

Answer 1

The angular momentum of the system can be calculated using the formula L = I * π. When the stick is pivoted about an axis passing through its center, the angular momentum is 3.02 kg*m^2/s. When the stick is pivoted about an axis at the end furthest from the attached mass, the angular momentum is 15.62 kg*m^2/s.

Step-by-step explanation:

The angular momentum of a system is given by the formula L = I * π, where L is the angular momentum, I is the moment of inertia, and π is the angular velocity.

(a) To find the angular momentum of the system when the stick is pivoted about an axis passing through its center, we need to calculate the moment of inertia of the stick and the attached mass. The moment of inertia of the stick can be calculated using the formula I = (1/3) * m * L^2, where m is the mass of the stick and L is its length. Substituting the values, we get: I = (1/3) * 0.225 kg * (2 m)^2 = 0.3 kg*m^2. The moment of inertia of the attached mass can be calculated using the formula I = m * r^2, where m is the mass of the attached mass and r is its distance from the axis of rotation. Substituting the values, we get: I = 0.5 kg * (0.6 m)^2 = 0.18 kg*m^2. The total moment of inertia of the system is the sum of the moment of inertia of the stick and the attached mass: I_total = I_stick + I_attached = 0.3 kg*m^2 + 0.18 kg*m^2 = 0.48 kg*m^2. Finally, substituting the values into the formula for angular momentum, we get: L = I_total * π = 0.48 kg*m^2 * 6.30 rad/s = 3.02 kg*m^2/s.

(b) To find the angular momentum of the system when the stick is pivoted about an axis at the end furthest from the attached mass, we need to calculate the new moment of inertia. The moment of inertia of the stick remains the same as before: I_stick = 0.3 kg*m^2. The moment of inertia of the attached mass can be calculated using the parallel axis theorem, which states that the moment of inertia of an object about an axis parallel to and a distance d away from an axis through its center of mass is given by I = I_cm + m * d^2, where I_cm is the moment of inertia about the center of mass. In this case, the distance d is the length of the stick: d = 2 m. Let's calculate I_cm using the formula for a point mass: I_cm = m * r^2 = 0.5 kg * (0.6 m)^2 = 0.18 kg*m^2. Substituting the values, we get: I_attached = I_cm + m * d^2 = 0.18 kg*m^2 + 0.5 kg * (2 m)^2 = 0.18 kg*m^2 + 2 kg*m^2 = 2.18 kg*m^2. The total moment of inertia of the system is the sum of the moment of inertia of the stick and the attached mass: I_total = I_stick + I_attached = 0.3 kg*m^2 + 2.18 kg*m^2 = 2.48 kg*m^2. Finally, substituting the values into the formula for angular momentum, we get: L = I_total * π = 2.48 kg*m^2 * 6.30 rad/s = 15.62 kg*m^2/s.

Answer 2

(a) The angular momentum of the system when the stick is pivoted about an axis perpendicular to the table and passing through its center is
`\(3.024 \, \text{kg} \cdot \text{m}^2/\text{s}\).`

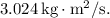
(b) The angular momentum of the system when the stick is pivoted about an axis perpendicular to it and at the end furthest from the attached mass is
`\(1.89 \, \text{kg} \cdot \text{m}^2/\text{s}\).`

(a) When the stick is pivoted about an axis perpendicular to the table and passing through its center:

The formula for angular momentum is
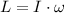`\(L = I \cdot \omega\)`, where L is the angular momentum, I is the moment of inertia, and
`\(\omega\)` is the angular speed.

First, let's calculate the moment of inertia of the system about the center when pivoted from its center.

The moment of inertia I for a point mass rotating about an axis at a distance r is
`\(I = m \cdot r^2\).`

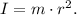
Given:

Mass of the stick
`(\(m_{\text{stick}}\)) = 0.225 kg`

Length of the stick
`(\(L_{\text{stick}}\)) = 2.0 m`

Distance of the attached mass from the center
`(\(r_{\text{attached}}\)) = 60.0 cm = 0.6 m`

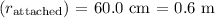
Angular speed
`(\(\omega\)) = 6.30 rad/s`

First, calculate the moment of inertia of the stick about its center:

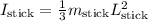`\(I_{\text{stick}} = (1)/(3) m_{\text{stick}} L_{\text{stick}}^2\)` (for a thin rod rotating about its center)

`\(I_{\text{stick}} = (1)/(3) * 0.225 \, \text{kg} * (2.0 \, \text{m})^2 = 0.30 \, \text{kg} \cdot \text{m}^2\)`

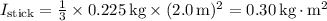
The moment of inertia of the attached mass about the center is
`\(I_{\text{attached}} = m_{\text{attached}} * r_{\text{attached}}^2\):`

`\(I_{\text{attached}} = 0.500 \, \text{kg} * (0.6 \, \text{m})^2 = 0.18 \, \text{kg} \cdot \text{m}^2\)`

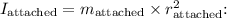
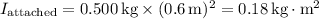
The total moment of inertia about the center is the sum of the moments of inertia of the stick and the attached mass:

`\(I_{\text{total}} = I_{\text{stick}} + I_{\text{attached}} = 0.30 \, \text{kg} \cdot \text{m}^2 + 0.18 \, \text{kg} \cdot \text{m}^2 = 0.48 \, \text{kg} \cdot \text{m}^2\)`

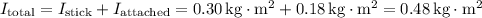
Now, calculate the angular momentum using the formula
`\(L = I \cdot \omega\):`

`\(L_{\text{total}} = I_{\text{total}} \cdot \omega = 0.48 \, \text{kg} \cdot \text{m}^2 * 6.30 \, \text{rad/s} = 3.024 \, \text{kg} \cdot \text{m}^2/\text{s}\)`

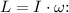
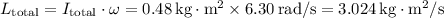
(b) When the stick is pivoted about an axis perpendicular to it and at the end furthest from the attached mass:

In this case, the moment of inertia will be different because the pivot point changes.

For a thin rod rotating about one end, the moment of inertia
`\(I_{\text{end}} = (1)/(3) m_{\text{stick}} L_{\text{stick}}^2\).`

`\(I_{\text{end}} = (1)/(3) * 0.225 \, \text{kg} * (2.0 \, \text{m})^2 = 0.30 \, \text{kg} \cdot \text{m}^2\)`

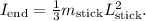
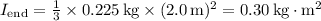
The total moment of inertia about this pivot point is only considering the stick itself, as the point mass is at the pivot:

`\(I_{\text{total, end}} = I_{\text{end}} = 0.30 \, \text{kg} \cdot \text{m}^2\)`

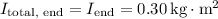
Using the same angular speed, calculate the angular momentum:

`\(L_{\text{total, end}} = I_{\text{total, end}} \cdot \omega = 0.30 \, \text{kg} \cdot \text{m}^2 * 6.30 \, \text{rad/s} = 1.89 \, \text{kg} \cdot \text{m}^2/\text{s}\)`