Question

Stephen has just purchased a home for ​$130,300. A mortgage company has approved his loan application for a​ 30-year fixed-rate loan at ​5.25%. Stephen has agreed to pay ​20% of the purchase price as a down payment. If Stephen made the same loan for 20​ years, how much interest would he​ save?

Answer 1

Answer: Stephen would save approximately $38,642.53 in interest by taking a 20-year loan instead of a 30-year loan

Explanation:

To determine how much interest Stephen would save by taking a 20-year loan instead of a 30-year loan, we need to follow these steps:

1. Calculate the loan amount after the down payment.

2. Calculate the monthly payment for both the 30-year and 20-year loans using the formula for monthly payments on a fixed-rate mortgage.

3. Calculate the total interest paid for both loan terms.

4. Subtract the total interest of the 20-year loan from the 30-year loan to find the savings.

Step 1: Calculate the loan amount after the down payment

Down payment = 20% of $130,300

`\[ \text{Down payment} = 0.20 * 130,300 \]`

Loan amount = Purchase price - Down payment

`\[ \text{Loan amount} = 130,300 - \text{Down payment} \]`

Step 2: Calculate the monthly payment

The formula for monthly payments
`\( M \)` on a fixed-rate mortgage is:

`\[ M = P * (r(1+r)^n)/((1+r)^n-1) \]`

Where:

-
`\( P \)` is the principal loan amount

-
`\( r \)` is the monthly interest rate (annual rate divided by 12)

-
`\( n \)` is the total number of payments (number of years multiplied by 12)

We'll calculate \( M \) for both 30 years (n = 360) and 20 years (n = 240).

Step 3: Calculate the total interest paid

Total interest =
`\( n * M - P \)`

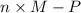
Step 4: Find the savings

Savings = Total interest (30 years) - Total interest (20 years)

Let's plug in the numbers and calculate.

Stephen would save approximately $38,642.53 in interest by taking a 20-year loan instead of a 30-year loan.