Question

Solve the system of equations using substitution. −2x−3y=19/y=−1

Answer 1

x = -8 and y = -1.

Explanation:

To solve the system of equations using substitution, we'll substitute the value of y from the second equation into the first equation.

Given system of equations:

`\sf -2x - 3y = 19 .......[i]`

`\sf y = -1 ...................[ii]`

Substitute y = -1 into the first equation:

`\sf -2x - 3(-1) = 19`

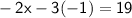
Simplify and solve for x:

`\sf -2x + 3 = 19`

`\sf -2x = 19 - 3`

`\sf -2x = 16`

`\sf x =( 16 )/(-2)`

`\sf x = -8`

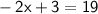
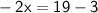
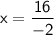
So, the solution to the system of equations is x = -8 and y = -1.