Question

− 3 ( 5 − 6 ) = 3 − 10 solve the equation

Answer 1

→ x - 3 (5x - 6) = 3 - 10x

→ x - 15x + 18 = 3 - 10x

→ -14x + 18 = 3 - 10x

→ -14x + 10x = 3 - 18

→ -4x = -15

4x = 15

x = 15/4

Answer 2

Question: x-3 (5x-6) = 3-10x

Answer:

`(11+/-√(-779) )/(30) i`

Explanation:

1. First start by applying PEMDAS. Multiply x-3 to the terms in the parentheses and be mindful of the signs,
2. After multiplying the expression, x-3 to the terms in the parentheses, it becomes clear that there is a quadratic forming on the left-hand side equal to the expression 3-10x.
3. Move 3-10x over to the left-hand side of the equation. Be mindful of the signs again, and add & subtract like-terms.
4. We are then left with
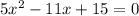   `5x^(2) -11x+15=0`. Because there are no common factors, we will need to utilize the quadratic formula to find the roots of x.
5. 
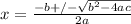   `x = \frac{-b+/-\sqrt{b^(2) -4ac} }{2a}` is the quadratic formula where 5 is a, -11 is b, and 15 is c.
6. When we plug the values into the formula, we are left with
   `x = (11+/-√( -779) )/(30)`.
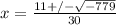7. By utilizing the discriminant technique (the portion of the formula within the square root), we find that -179 < 0.
   `b^(2) -4ac < 0.\\-11^(2) -4(5)(15) = -179 < 0.`
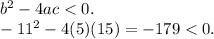
8. Because -179 is less than 0, we know that the roots for this equation are complex roots, i, thus the final answer is
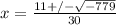   `x = (11+/-√( -779) )/(30)`i.

Please see the attached photo.

Please let me know if this helped!!!