Question

Solve for x.
A. 7
B. 3
C. 4
D. 5

Answer 1

• D. 5

Explanation:

`\sf (9)(9+x)=(7)(7+11)`

Simplify:-

`\sf (9)(9+x)=7(7+11)`

`\sf (9)(9+x)=7*18`

`\sf (9)(9+x)=126`

Divide both sides by 9:-

`\sf \cfrac{(9)(9+x)}{9} =\cfrac{126}{9}`

`\sf 9+x=14`

`\sf x+9=14`

Subtract both sides by 9 :-

`\sf x+9-9=14-9`

`\sf x= 5`

Therefore x = 5.

_______________________

Hope this helps!

Have a great day!

Answer 2

`\huge\boxed{\sf x = 5}`

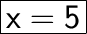
Explanation:

Secant-secant theorem:

According to secant-secant theorem, the product of a secant segment and its external segment is equal to the product of other secant segment and external segment.

Solution:

According to the statement,

9(9 + x) = 7(7 + 11)

• Distribute.

81 + 9x = 7(18)

81 + 9x = 126

• Subtract 81 from both sides.

9x = 126 - 81

9x = 45

• Divide both sides by 9.

x = 45/9

x = 5

NOTE: Refer to the attached file for more understanding of the topic.

`\rule[225]{225}{2}`