Answer:
b = 4
Explanation:
the equation of a line in slope- intercept form is
y = mx + c ( m is the slope and c the y- intercept )
given
2x - 5y = 7 ( subtract 2x from both sides )
- 5y = - 2x + 7 ( divide through by - 5 )
y =
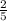 x -
x -
 ← in slope- intercept form
← in slope- intercept form
with slope m =
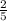
given a line with slope m then the slope of a line perpendicular to it is
 = -
= -
 = -
= -
 = -
= -
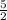
given
10x + by = 7 is perpendicular to the line 2x - 5y = 7
then line 10x + by = 7 has a slope m of -
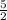
subtract 10x from both sides
by = - 10x + 7 ( divide through by b , b ≠ 0 )
y = -
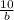 x +
x +
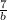 ← in slope- intercept form
← in slope- intercept form
with slope m = -
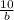
equate -
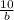 and -
and -
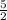 and solve for b
and solve for b
-
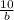 = -
= -
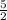 ( cross- multiply )
( cross- multiply )
5b =- 2 × - 10 = 20 ( divide both sides by 5 )
b = 4