Question

A barefoot field-goal kicker imparts a speed of 30 m/s to a football at rest. If the football has a mass of 0.45 kg and time of contact with the football is 0.035 s, what is the force exerted on the foot?

Answer 1

Approximately
`386\; {\rm N}`.

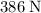Step-by-step explanation:

To find the average force on the foot, make use of the fact that impulse is equal to the change in momentum. Start by finding the change in the momentum of the football from the initial and final velocity- this change in momentum would be equal to the impulse on the football. Divide the impulse by the duration of the motion to find the average force exerted.

Multiply the mass of the football
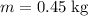`m = 0.45\; {\rm kg}` by velocity to find the momentum of this football.

Since the football was initially at rest with a velocity of
`u = 0\; {\rm m\cdot s^(-1)}`, initial momentum would be
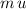`m\, u`. At a velocity of
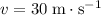`v = 30\; {\rm m\cdot s^(-1)}`, the momentum of this football would be
`m\, v`.

Subtract the initial value of momentum from the new value to find the change in momentum:

`\Delta p = m\, (v - u)`.

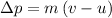Assuming that friction on the football is negligible. The impulse
`J` the person exerted on the football would be equal to the change in the momentum of the football:

`J = \Delta p = m\, (v - u)`.

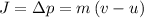Divide impulse by the duration of the motion
`\Delta t` to find the average force that the person exerted on the football:

`\begin{aligned}F &= (J)/(\Delta t) \\ &= (m\, (v - u))/(\Delta t)\\ &= ((0.45)\, (30 - 0))/(0.035)\; {\rm kg\cdot m\cdot s^(-2)} \\ &\approx 386\; {\rm N}\end{aligned}`.

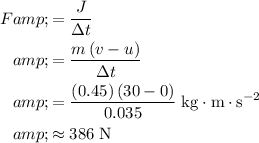By Newton's Laws of Motion, the reaction force that the football exerts on the person would be of the same magnitude: approximately
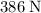`386\; {\rm N}`.