Question

Show that the point A(a,b+c) , B(b, c+a) , C(c, a+b) lie on a straight line

Answer 1

see explanation

Explanation:

if the points lie on a straight line then the slopes m between consecutive points will be equal, that is

`m_(AB)` =
`m_(BC)`

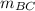
calculate slopes using the slope formula

m =
`(y_(2)-y_(1) )/(x_(2)-x_(1) )`

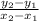
with (x₁, y₁ ) = A (a, b+c ) and (x₂, y₂ ) = B (b, c+a )

`m_(AB)` =
`(c+a-b-c)/(b-a)` =
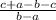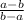`(a-b)/(b-a)` ← factor out - 1 from each term on the denominator

`m_(AB)` =
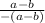`(a-b)/(-(a-b))` ← cancel out (a - b) on numerator/ denominator

`m_(AB)` =
`(1)/(-1)` = - 1

similarly

with (x₁, y₁ ) = B (b, c + a ) and (x₂, y₂ ) = C (c, a + b )

`m_(BC)` =
`(a+b-c-a)/(c-b)` =
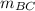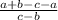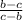`(b-c)/(c-b)` ← factor out - 1 from each term on the denominator

`m_(BC)` =
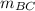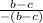`(b-c)/(-(b-c))` ← cancel out (b - c ) on numerator/ denominator

`m_(BC)` =
`(1)/(-1)` = - 1

since
`m_(AB)` =
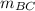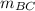`m_(BC)` = - 1

then the points are collinear , lie on straight line