Question

On the coordinate plane, point T is located at (0,7) and point U is located at (x,-5). The distance between points T and U is 15 units.

What are the two possible values of x?

Answer 1

x = 9 or x = -9

Explanation:

The parameters of the given points are;

The location of the point T = (0, 7)

The location of the point U = (x, -5)

Then distance between points T and U = 15 units

The distance between points A and B, 'd', given their coordinates, A(x₁, y₁) and B(x₂, y₂) is found using the following formula;

`d=\sqrt{\left (y_(2)-y_(1) \right )^(2)+\left (x_(2)-x_(1) \right )^(2)}`

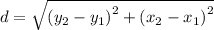
Therefore, for the given points, T(0, 7) and U(x, -5), we get;

`15 = \sqrt{\left ((-5) - 7 \right )^(2)+\left (x - 0 \right )^(2)}`

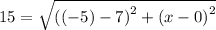
Squaring both sides, gives;

`15^2 = \left ((-5) - 7 \right )^(2)+\left (x - 0 \right )^(2)} = (-12)^2 + x^2`

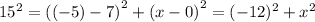
∴ x² = 15² - (-12)² = 81 = 9²

x² = 9²

∴ x = ±√(9²) = ± 9

The possible values of x are x = 9 or x = -9