Question

Find the exact values below

cot7pie/4



Sec 7pie/4

Answer 1

-1

`√(2)`

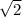
Explanation:

We can find the exact trigonometric values using the unit circle.

Cotangent

We are first asked about cot. This is the abbreviation of cotangent. Cotangent is the reciprocal of tangent (tan). So, in order to solve for cot, we first need to find tan. To find the exact value of tan (7π/4), we need to use the unit circle.

By looking at the unit circle, we can tell that the tangent of 7π/4 = -1. If you cannot find tangent on a unit circle remember that tan = sin/cos. Then, we can take the reciprocal. Since the reciprocal of -1 is -1, cot(7π/4) = -1.

Secant

The question asks for sec, which is the abbreviation of secant. Secant is the reciprocal of cosine (cos). If we want to find secant, we need to find cos first. Once again, we should use the unit circle to find the exact value.

If we look for the cos of 7π/4 on the unit circle we can find that cos(7π/4) =
`(√(2) )/(2)`. Then, we need to take the reciprocal. The reciprocal of
`(√(2) )/(2)` is
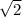`√(2)`. This means that sec(7π/4) = √2.