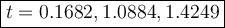Question

NO LINKS!! URGENT HELP PLEASE!!

Please help me with this solution Part 4​

Answer 1

d) t = 0.1682, 1.0884, 1.425

`\hrulefill`

Explanation:

To find the solutions to the equation 3 cos(5t) = 2 in the interval 0 ≤ t ≤ π/2 radians, first isolate the cosine term and then solve for t.

Isolate the cosine term by dividing both sides of the equation by 3:

`\begin{aligned}3 \cos (5t)&=2\\\\\cos (5t)&=(2)/(3)\end{aligned}`

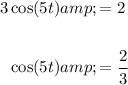
Solve for 5t by taking the arccosine of both sides of the equation, remembering that the cosine function has a periodicity of 2π, and that the cosine function is positive in quadrants I and IV:

`\begin{aligned}\arccos\left(\cos(5t)\right)&=\arccos\let((2)/(3)\right)\\\\5t&=0.84106867...+2\pi n,\;2\pi-0.84106867...+2\pi n\end{aligned}`

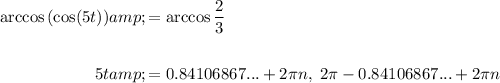
Finally, divide both sides of the equation by 5 to solve for t:

`\begin{aligned}(5t)/(5)&=(0.84106867...)/(5)+(2\pi n)/(5),\;(2\pi-0.84106867...)/(5)+(2\pi n)/(5)\\\\t&=0.16821373...+(2\pi)/(5)n, 1.08842332...+(2\pi)/(5)n\end{aligned}`

The given interval is 0 ≤ t ≤ π/2 which is approximately 0 ≤ t ≤ 1.5708.

To find the solutions for t that are in the given interval, add integer multiples of 2π/5 to the found solutions of t.

`t=0.1682`

`t=0.1682+(2\pi)/(5)=1.4249`

`t=1.0884`

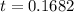
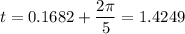
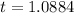
Therefore, the solutions of 2cos(5t) = 2 in the given interval are:

`\large\boxed{t=0.1682, 1.0884,1.4249}`