Question

PLS PLS PLS HELP !!! -25 POINTS-

Answer 1

7

`7 - \alpha \tan(39.7 = y)`

Explanation:

is that correct

Answer 2

The shaded area is exactly 27π cm², or approximately 84.8 cm² (rounded to the nearest tenth).

Explanation:

The given diagram shows circle O with a radius of 6 cm, with section AOB removed. The central angle of the removed section is 90°.

Angles around a point sum to 360°. Therefore, as 90° is one-quarter of 360°, the removed section AOB is one quarter of the area of circle O. Therefore, the shaded area is 3/4 of the area of the circle.

The formula for the area of a circle is A = πr², where r is the radius of the circle.

To find the shaded area, multiply the area of circle O by 3/4:

`\begin{aligned}\sf Shaded\;Area&=(3)/(4)\cdot \sf Area\;of\;circle\;O\\\\&=(3)/(4) \cdot \pi (6)^2\\\\&=(3)/(4) \cdot \pi (36)\\\\&=27\pi \\\\&=84.8230016...\\\\&=84.8\; \sf cm^2\;(nearest\;tenth)\end{aligned}`

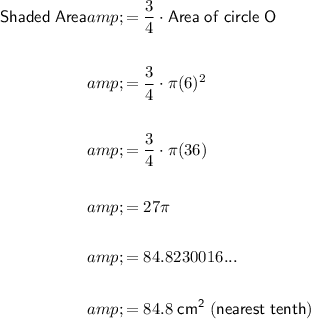
Therefore, the shaded area is exactly 27π cm², or approximately 84.8 cm² (rounded to the nearest tenth).