Question

NO LINKS!! URGENT HELP PLEASE!! Find the measure of line segment MK​

Answer 1

MK = 21

Explanation:

8( 8+x+5)= 7(7+2x+1)

8(x+13)=7(2x+8)

8x+104=14x+56

8x-14x=56-104

-6x=-48

x=8

MK = 8+5+8=21

Answer 2

`\overline{\sf MK}=21`

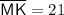
Explanation:

The given diagram shows two secant segments MK and CK that intersect at the exterior point K. Their external parts are LK and JK, respectively.

To find the measure of line segment MK, we first need to find the value of x. To do this, we can use the Intersecting Secants Theorem.

The Intersecting Secants Theorem states that the product of the measures of one secant segment and its external part is equal to the product of the measures of the other secant segment and its external part.

Therefore:

`\sf \overline{MK} \cdot \overline{LK} =\overline{CK} \cdot \overline{JK}`

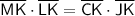
Substitute the value (or expression) of each line segment into the equation and solve for x:

`\begin{aligned}(x+5+8) \cdot 8&=(2x+1+7) \cdot 7\\(x+13)\cdot8&=(2x+8)\cdot7\\8x+104&=14x+56\\48&=6x\\8&=x\end{aligned}`

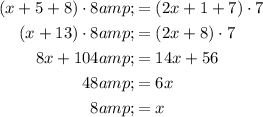
Therefore, x = 8.

Substitute the found value of x into the expression for line segment MK:

`\begin{aligned}\overline{\sf MK}&=x+5+8\\&=8+5+8\\&=21\end{aligned}`

Therefore, the measure of line segment MK is 21 units.