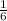Answer:
x >
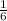
Explanation:
- 2(8x - 4) < 2x + 5 ← distribute parenthesis on left side
- 16x + 8 < 2x + 5 ( subtract 2x from both sides )
- 18x + 8 < 5 ( subtract 8 from both sides )
- 18x < - 3
divide both sides by - 18, reversing the symbol as a result of dividing by a negative quantity.
 x >
x >
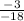 , that is
, that is
x >