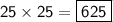Hello!
Answer:
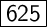
Explanation:
Let x and y be the two numbers.
So we have this equation:
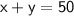
We want to maximize xy. The question translates to finding the maximum of the function f defined by:
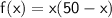
Simplify the function:

The graph of the function f is a concave down parabola. The maximum of f occurs at the vertex.
To find the vertex, we equate
 to 0, and we get:
to 0, and we get:
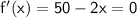 if only
if only
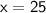
Using the first equation, we get:
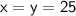
So the maximum product is: