Question

The linear system x-y= -5 and x+y=1 has how many solutions

Answer 1

one solution

Explanation:

In order to determine the number of solutions for the given linear system, we can solve it using elimination or substitution method. Let's use the elimination method to solve the system:

Given system:

1) x - y = -5

2) x + y = 1

Adding equation (1) and equation (2), we eliminate the variable 'y':

(x - y) + (x + y) = -5 + 1

2x = -4

`\tt x =-( 4)/(2)`

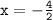
x = -2

Now, substitute the value of x into either equation (1) or equation (2). Let's use equation (1):

x - y = -5

-2 - y = -5

-y = -5 + 2

-y = -3

y = 3

Thus, we have found a unique solution for the system: x = -2 and y = 3.

Therefore, the given linear system has exactly one solution.