Answer:
C) y = -3
Explanation:
In order to find the equation of a line satisfying the given conditions, we'll use the point-slope form of a line, which is given by:
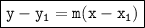
Where (x1, y1) is a point on the line and m is the slope of the line.
In this case,
we have a line perpendicular to x = -7, which is a vertical line. The slope of a vertical line is undefined.
Therefore, the slope of the line perpendicular to it is 0.
We are given that the line passes through the point (2, -3).
Plugging these values into the point-slope form, we have:
y - (-3) = 0(x - 2)
y + 3 = 0
y = -3
Therefore, the equation of the line satisfying the given conditions is:
y = -3
So the correct option is: C) y = -3