Answer:
$422.73
Explanation:
To calculate how much would you have to deposit in an account with a 8% interest rate, compounded monthly, to have $800 in your account 8 years later, use the compound interest formula.
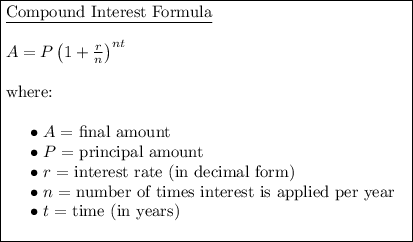
Given values:
- A = $800
- r = 8% = 0.08
- n = 12 (monthly)
- t = 8 years
Substitute the given values into the formula and solve for P:
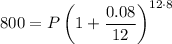

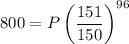

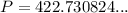

Therefore, you would have to deposit $422.73 in an account with a 8% interest rate, compounded monthly, to have $800 in your account 8 years later.