Question

What are the vertex and range of y = |x + 2| − 6?

Answer 1

Vertex = (-2, -6)

Range = y ≥ -6

Explanation:

The method of finding the vertex and range is the same as finding the vertex and range of parabolas. The vertex of a|x - h| + k is (h, k).

But suppose we follow the definition:

`\displaystyle{|x+a| = \begin{cases} x+a \ \ \ \ \ x \geq -a \\\\ -x-a \ \ \ \ \ x < -a \end{cases}}`

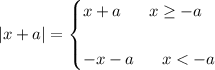
Then we have. for x ≥ -2,

y = x + 2 - 6

y = x - 4

And we have, for x < -2,

y = -x - 2 - 6

y = -x - 8

Before we find the vertex, we have to check the range first. Since the function y = |x + 2| - 6.

y + 6 = |x + 2|

In this case, y + 6 has to be greater or equal to 0 because the right side of the equation is in absolute terms. Therefore, y + 6 ≥ 0 becomes y ≥ -6. Thus, the range is y ≥ -6.

To find the vertex, we refer to the range. The minimum value must be -6 because that's the minimum point. Substitute y = -6 in the equation and solve for x-value:

-6 = |x + 2| - 6

-6 + 6 = |x + 2|

0 = |x + 2|

0 = x + 2

x = -2

Therefore, the vertex is at (-2, -6).