The rate of change of the function
 at
at
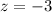 , to the nearest tenth, is 154.0.
, to the nearest tenth, is 154.0.
The instantaneous rate of change of the function
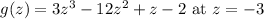 can be found by taking the derivative of
can be found by taking the derivative of
 with respect to
with respect to
 and evaluating it at
and evaluating it at
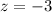 .
.
The derivative of
 , denoted as
, denoted as
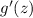 , is
, is
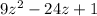 . Evaluating this at
. Evaluating this at
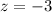 gives us the instantaneous rate of change:
gives us the instantaneous rate of change:
![\[ g'(-3) = 9(-3)^2 - 24(-3) + 1 = 154 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/xvrrbus5yigqg19e4dxsl9l17dy9roh1gf.png)
This is the exact value of the instantaneous rate of change at
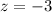 .
.
However, to approximate this value using an interval of 0.001, we calculate the average rate of change between
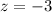 and
and
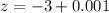 . This gives us an approximate value of:
. This gives us an approximate value of:
![\[ (g(-3 + 0.001) - g(-3))/(0.001) \approx 153.961 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/c8pk2qrcpbwx593vgukk8fcutl2muwo8nb.png)
Rounded to the nearest tenth, the approximate instantaneous rate of change is 154.0 (since the exact and the approximate values are very close, the rounding doesn't change the result).
Therefore, The answer is 154.0.