Question

50 PTS!!! P L Z!!!!

Choose the correct answer.

Average speed of Car 1 = 35 mph.
Average speed of Car 2 = 55 mph.
Time elapsed between start of Car 1 and start of Car 2 = 18 minutes.

How long before Car 2 overtakes Car 1?
0.53
0.43
0.33
hour.

Answer 1

0.53 hours

Explanation:

Given values:

• The average speed of Car 1 is 35 mph.
• The average speed of Car 2 is 55 mph.
• The time elapsed between the start of Car 1 and the start of Car 2 is 18 minutes (0.3 hours).

Let t be the time (in hours) that it takes Car 2 to overtake Car 1.

If the time elapsed between the start of Car 1 and the start of Car 2 is 0.3 hours, then the time for Car 1 is (t + 0.3).

Speed-Distance-Time formula

`\boxed{\sf Speed=(Distance)/(Time)}`

Using the Speed-Distance-Time formula, we can create two equations for the distance each car travels:

`\textsf{Car\;1:} \quad d=35 * (t+0.3)= 35t+10.5`

`\textsf{Car\;2:} \quad d=55 * t = 55t`

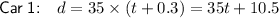
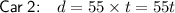
As the distance that both cars travel is the same, substitute one equation into the other and solve for t:

`\begin{aligned}55t&=35t+10.5\\55t-35t&=35t+10.5-35t\\20t&=10.5\\20t / 20&=10.5 / 20\\t&=0.525\\t&=0.53\; \sf hours\;(nearest\;hundredth)\end{aligned}`

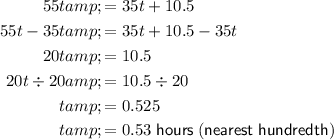
Therefore, it will take 0.53 hours before Car 2 overtakes Car 1.