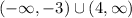Question

X² -x > 12
How to solve the inequality and turn into a interval notation ? Step by step

Answer 1

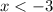`x < -3` or
`x > 4`

Explanation:

Trickier Method (but more direct)

`x^2-x > 12\\x^2-x+0.25 > 12.25\\(x-0.5)^2 > 12.25\\|x-0.5| > 3.5\\\\x-0.5 > 3.5\\x > 4\\\\-(x-0.5) > 3.5\\x-0.5 < -3.5\\x < -3`

Easier Method (test values required)

`x^2-x > 12\\x^2-x-12 > 0\\(x-4)(x+3) > 0`

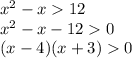
Since
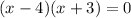`(x-4)(x+3)=0` has the solutions
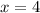`x=4` and
`x=-3`, then either all possible solutions to the original inequality are between 4 and -3, or outside of that.

If we test a value such as
`x=3` for example, notice the following:

`(3-4)(3+3)\stackrel{?}{ > }0\\(-1)(6)\stackrel{?}{ > }0\\-6\\gtr0`

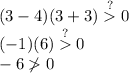
This tells us that all possible solutions are not between 4 and -3, therefore,
`x > 4` or
`x < -3`.

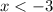In interval notation, this would be
`(-\infty,-3)\cup(4,\infty)`