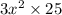Question

Simplify. multiply and remove all perfect squares from inside the square roots. assume x xx is positive. 3 x 4 ⋅ 5 x 2 ⋅ 10 = 3x 4 ​ ⋅ 5x 2 ​ ⋅ 10

Answer 1

The simplified expression without any perfect squares inside the square roots is
`3x^2 * 25.`

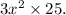
To simplify the expression and remove all perfect squares from inside the square roots, we can apply basic rules of exponents and factorization.

Starting with the given expression:

`3x^4 * 5x^2 * 10`

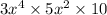
First, we can break down 10 into its prime factors: 10 = 2 * 5.

Next, we look for perfect squares among the variables' exponents.
`x^4` and
`x^2` are perfect squares since they can be expressed as
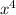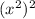`(x^2)^2` and x^2, respectively.

Now, we rewrite the expression, factoring out the perfect squares:

`3x^4 * 5x^2 * 10 = 3 * (x^2)^2 * 5 * x^2 * (2 * 5)`

Now, we simplify the expression further:

=
`3 * x^2 * 5 * (2 * 5)`

=
`3 * x^2 * 25`

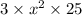
Finally, we rewrite 25 as a perfect square:
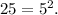`25 = 5^2.` Now we have:

=
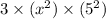
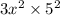
`3 * (x^2) * (5^2)`

=
`3x^2 *5^2`

=
`3x^2 * 25`